The Distance Around a Circle is Called Circumference
The distance around the outside of the circle is called the circumference.
Distance Around a Circle – Circumference Quiz
Instructions: Calculate the distance around a circle in the exercises that follow. You may want to see the formulas and examples in the next section first.
[WpProQuiz 36]
Circumference – Illustrated
Look at the illustration of a circle below:
The distance of the black line around the outside of a circle like the one above is its circumference.
Formula for Circumference of a Circle
You will need to remember the symbol π when using the formula for circumference of a circle.
Circumference = 2πr
The radius of the circle is represented by r in the above formula.
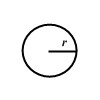
What is the Radius?
The radius is the measurement from the center of a circle to the outside edge of the circle.
The circle below illustrates a radius:
Circumference with Diameter
Since the radius of the circle is half of the diameter, you can also use this formula for circumference of a circle:
Circumference = πd
The diameter of the circle is represented by d in the above formula.
Questions on the Circumference of a Circle
Values for π
You can sometimes express circumference as a number and the π symbol.
Other times, you may be asked to use a value for π, such as 3.14
Practical Problems
Some questions on the circumference of a circle may also involve practical situations, like measuring the distance around the outside of a wheel or other circular object.
Difference in Circumference
You may also see questions that ask you to find the difference between the circumferences of two circles.
Other Aspects of Circles
Area
Area is a measurement of the space on the inside of a circle.
Chords
Chords are lines that are drawn between two points on the outside of a circle.
Arcs
An arc is a part of the circumference of a circle.
Semicircles
A semicircle is half of a circle. You will need to understand semicircles for problems on hybrid shapes.
We will look at area, chords, arcs, and hybrid shapes in separate posts.